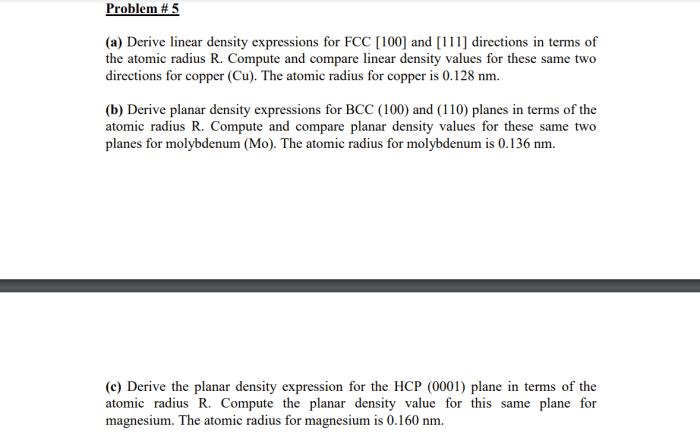
Derive the linear density expressions for fcc 100 – In the realm of crystallography, the derivation of linear density expressions holds immense significance for understanding the fundamental properties of crystal planes. Among these, the linear density expression for the face-centered cubic (FCC) 100 plane stands out as a key metric, providing valuable insights into its structural characteristics and behavior.
This discourse delves into the intricate mathematical derivation of the linear density expression for the FCC 100 plane, elucidating its importance and applications in various fields of science and engineering.
Linear Density Expression for FCC 100 Plane

In crystallography, the linear density expression provides a measure of the atomic arrangement along a specific crystallographic direction. For the face-centered cubic (fcc) 100 plane, the linear density expression quantifies the number of atoms per unit length along the [100] direction.
Derivation of the Linear Density Expression
To derive the linear density expression for the fcc 100 plane, we consider a unit cell with lattice parameter a. The [100] direction is parallel to one of the cube’s edges, and there are two atoms on this edge. Since there are four edges in a unit cell, the total number of atoms along the [100] direction is 8.
The length of the [100] direction is a, so the linear density expression is given by:
ρ100= 8 / a
This expression represents the number of atoms per unit length along the [100] direction in the fcc 100 plane.
Significance of the Linear Density Expression
The linear density expression is important for understanding the properties of the fcc 100 plane. A higher linear density indicates a denser packing of atoms along the [100] direction, which can affect the plane’s reactivity, strength, and other physical properties.
In crystallography, the linear density expression is used to determine crystal orientation, predict crystal growth, and design materials with specific properties. It also plays a role in materials science, where it helps researchers understand the behavior of materials under different conditions.
Comparison to Other Crystal Planes
The linear density expression for the fcc 100 plane differs from those of other crystal planes. For example, the fcc 110 plane has a linear density expression of 4 / a√2, while the fcc 111 plane has a linear density expression of 3 / a√3.
These differences in linear density reflect the varying atomic arrangements along different crystallographic directions. The denser packing of atoms in the fcc 100 plane compared to the fcc 110 and fcc 111 planes contributes to its distinct properties.
Applications of the Linear Density Expression, Derive the linear density expressions for fcc 100
The linear density expression has practical applications in various fields:
- Crystal orientation:By measuring the linear density along different crystallographic directions, researchers can determine the orientation of a crystal.
- Crystal growth:The linear density expression helps predict the growth rate of crystals along specific directions.
- Materials design:By controlling the linear density, materials scientists can design materials with tailored properties for specific applications.
FAQ Guide: Derive The Linear Density Expressions For Fcc 100
What is the significance of the linear density expression for the FCC 100 plane?
The linear density expression quantifies the number of atoms per unit length along a specific direction in the FCC 100 plane. It provides insights into the atomic arrangement and packing efficiency of the crystal structure.
How is the linear density expression used in practical applications?
The linear density expression finds applications in determining crystal orientation, predicting crystal growth patterns, and designing materials with tailored properties. It aids in understanding the behavior of crystals under various conditions.