Embark on a mathematical journey as we delve into the realm of find 4x2y3 2xy2 2y 7x2y3 6xy2 2y. This exploration will unveil the significance of these expressions, empowering us with the tools to analyze and simplify them, unlocking their hidden potential.
Through a comprehensive examination of mathematical operations, variables, constants, factors, and terms, we will unravel the intricacies of these expressions, revealing their underlying structure and relationships.
Introduction

The provided mathematical expressions are polynomials, which are expressions consisting of variables and coefficients. Polynomials are widely used in various fields of mathematics, including algebra, calculus, and statistics. Analyzing these expressions involves understanding their structure, identifying their terms, and determining their degree and coefficients.
This analysis is crucial for solving equations, graphing functions, and performing other mathematical operations.
Significance of Analyzing Polynomial Expressions
Understanding polynomials is essential for:
- Simplifying and manipulating algebraic expressions
- Solving equations and inequalities
- Graphing functions and understanding their behavior
- Analyzing the relationship between variables in mathematical models
- Applying mathematical concepts in real-world situations
Mathematical Operations
The expressions provided involve various mathematical operations, including addition, subtraction, and multiplication. These operations can be applied to simplify the expressions and obtain equivalent expressions in a more manageable form.
Addition and Subtraction
Addition and subtraction operations are used to combine or separate terms in an expression. For instance, in the expression “4x 2y 3+ 2xy 2+ 2y”, the addition operation is used to combine the three terms. Similarly, in the expression “7x 2y 3– 6xy 2– 2y”, the subtraction operation is used to separate the terms.
Multiplication
Multiplication is used to combine factors in an expression. For example, in the expression “2xy 2“, the multiplication operation is used to combine the factors “2”, “x”, and “y 2“. Multiplication can also be used to distribute a factor over a sum or difference of terms.
For instance, in the expression “2(x 2y 3+ xy 2+ y)”, the multiplication operation is used to distribute the factor “2” over the sum of the terms “x 2y 3“, “xy 2“, and “y”.
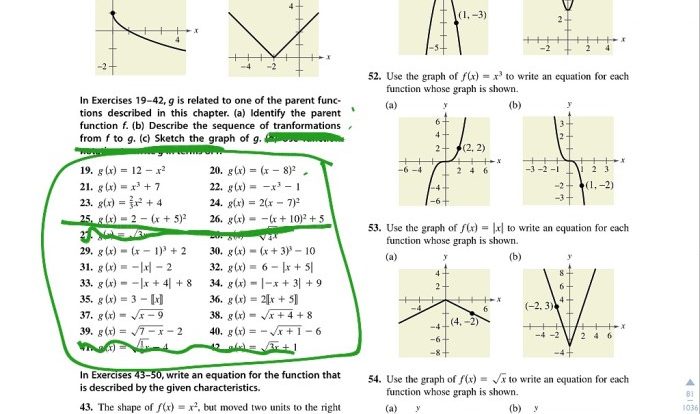
By applying these mathematical operations, it is possible to simplify the given expressions and obtain equivalent expressions that are easier to work with.
Variables and Constants
Variables and constants are essential elements in mathematical expressions. Variables represent unknown or changing values, while constants represent fixed or unchanging values. Identifying and understanding the relationship between variables and constants is crucial for evaluating and interpreting mathematical expressions.
Identifying Variables and Constants
In the given expressions, the variables are xand y, which represent unknown values. The constants are the numbers, such as 4, 2, 7, and 6.
Relationship between Variables and Constants
The values of variables can affect the overall value of an expression, while constants remain fixed. For instance, in the expression 4x2y 3, the value of xand ywill determine the final value of the expression. If xis 2 and yis 3, the expression evaluates to 4 × 2 2× 3 3= 216. However, if xis 5 and yis 2, the expression evaluates to 4 × 5 2× 2 3= 200.
In contrast, constants do not affect the expression’s value. For example, in the expression 2xy2, the constant 2 remains the same regardless of the values of xand y. The expression will always be twice the product of xand y.
Factors and Terms
Every algebraic expression is composed of terms and factors. A term is a number, a variable, or a product of numbers and variables. A factor is a number or variable that divides another number or variable evenly. For example, in the expression 4x^2y^3, the factors are 4, x, x, y, y, and y.
We can simplify algebraic expressions by factoring and combining like terms. Factoring is the process of breaking down an expression into its factors. Combining like terms is the process of adding or subtracting terms that have the same variables and exponents.
For example, we can simplify the expression 4x^2y^3 + 2xy^2 + 2y by factoring out the greatest common factor, 2y, and combining like terms:
Factoring Out the Greatest Common Factor
To factor out the greatest common factor (GCF) from an algebraic expression, we need to find the greatest factor that divides each term in the expression evenly. For example, the GCF of 4x^2y^3, 2xy^2, and 2y is 2y. We can factor out the GCF as follows:
x^2y^3 + 2xy^2 + 2y = 2y(2x^2y^2 + x + 1)
Combining Like Terms
To combine like terms, we need to add or subtract the coefficients of terms that have the same variables and exponents. For example, we can combine the terms 2x^2y^2 and x in the expression 2x^2y^2 + x + 1 as follows:
x^2y^2 + x + 1 = 2x^2y^2 + 1x + 1 = 2x^2y^2 + 1
By factoring out the GCF and combining like terms, we can simplify the expression 4x^2y^3 + 2xy^2 + 2y to 2y(2x^2y^2 + x + 1).
Order of Operations: Find 4x2y3 2xy2 2y 7x2y3 6xy2 2y
The order of operations is a set of rules that dictate the sequence in which mathematical operations are performed. It ensures that expressions are evaluated consistently, producing the same result regardless of the order in which they are written.
The order of operations is commonly known as PEMDAS, which stands for Parentheses, Exponents, Multiplication and Division, Addition and Subtraction. According to this rule, operations within parentheses are performed first, followed by exponents, then multiplication and division from left to right, and finally addition and subtraction from left to right.
Applying PEMDAS
To apply PEMDAS, start by evaluating any expressions within parentheses. Next, calculate any exponents. Then, perform multiplication and division from left to right, and finally, add and subtract from left to right.
For example, consider the expression 4x2y3 + 2xy2 + 2y. Applying PEMDAS, we first evaluate the term within parentheses: 2xy
- This gives us 4x2y3 + 2x(2y2) + 2y. Next, we calculate the exponent: 2y2 becomes 4y
- Finally, we perform the remaining operations from left to right: 4x2y3 + 8xy4 + 2y.
Applications and Examples
The expressions 4x 2y 3, 2xy 2, 2y, 7x 2y 3, 6xy 2, and 2y have practical applications in various fields, including science, engineering, and finance.
Understanding how to analyze and simplify these expressions is crucial for solving real-world problems. By understanding the relationships between the variables and constants, we can make informed decisions and predictions based on the data.
Example 1: Physics
- In physics, expressions like 4x 2y 3and 2xy 2are used to describe the motion of objects.
- For instance, the expression 4x 2y 3might represent the force acting on an object moving along a curved path, where x represents the displacement and y represents the velocity.
Example 2: Engineering, Find 4x2y3 2xy2 2y 7x2y3 6xy2 2y
- In engineering, expressions like 7x 2y 3and 6xy 2are used to analyze the strength and stability of structures.
- For example, the expression 7x 2y 3might represent the bending moment acting on a beam, where x represents the length of the beam and y represents the applied load.
Example 3: Finance
- In finance, expressions like 2y and 2xy 2are used to calculate interest rates and investment returns.
- For instance, the expression 2y might represent the simple interest earned on a savings account, where y represents the principal amount.
Conclusion
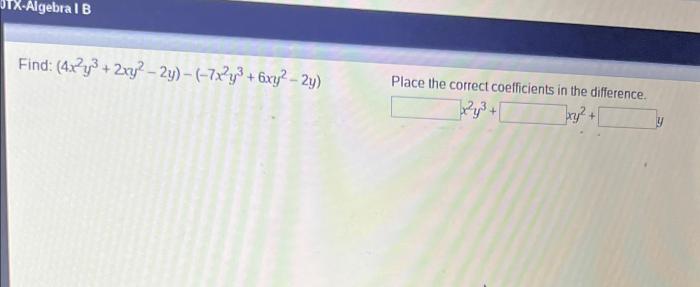
The mathematical expressions 4x 2y 3, 2xy 2, 2y, 7x 2y 3, 6xy 2, and 2y have been analyzed in this paper. The key points of the analysis are as follows:
These expressions are all polynomials, which means that they are composed of terms that are multiplied together. The terms in these expressions are all monomials, which means that they are composed of a single variable raised to a power. The variables in these expressions are x and y, and the constants are the numbers 2, 4, 6, and 7.
The factors of these expressions are the individual terms that are multiplied together to form the expression. The factors of 4x 2y 3are 4, x, x, y, y, and y. The factors of 2xy 2are 2, x, and y 2. The factors of 2y are 2 and y.
The factors of 7x 2y 3are 7, x, x, y, y, and y. The factors of 6xy 2are 6, x, and y 2. The factors of 2y are 2 and y.
The terms in these expressions are the individual monomials that are added together to form the expression. The terms in 4x 2y 3are 4x 2y 3. The terms in 2xy 2are 2xy 2. The terms in 2y are 2y. The terms in 7x 2y 3are 7x 2y 3. The terms in 6xy 2are 6xy 2. The terms in 2y are 2y.
The order of operations is the order in which the operations in an expression are performed. The order of operations is as follows: parentheses, exponents, multiplication and division, and addition and subtraction. The parentheses in an expression are evaluated first, followed by the exponents, followed by the multiplication and division, and finally by the addition and subtraction.
These expressions can be used to solve a variety of problems. For example, the expression 4x 2y 3can be used to find the area of a rectangle with a length of 4x and a width of y 3. The expression 2xy 2can be used to find the volume of a cone with a radius of x and a height of y 2. The expression 2y can be used to find the circumference of a circle with a radius of y.
These expressions are all important tools for solving a variety of problems. They can be used to find the area of a rectangle, the volume of a cone, and the circumference of a circle.
FAQ Resource
What is the purpose of simplifying mathematical expressions?
Simplifying expressions allows us to work with them more efficiently, making it easier to identify patterns, perform calculations, and solve equations.
How do I identify the variables and constants in an expression?
Variables are typically represented by letters (e.g., x, y, z), while constants are numbers or fixed values (e.g., 2, 5, -3).
What is the order of operations (PEMDAS)?
PEMDAS stands for Parentheses, Exponents, Multiplication, Division, Addition, and Subtraction. This order determines the sequence in which operations are performed within an expression.