Embark on a comprehensive exploration of practice 10-5 trigonometry and area, delving into the fundamental principles of trigonometry, area measurement, and their intertwined applications in real-world scenarios. This discourse unveils the captivating interplay between angles, trigonometric ratios, and the calculation of areas, equipping you with a robust understanding of these indispensable concepts.
Through a series of engaging practice problems, interactive exercises, and practical examples, we will illuminate the practical significance of trigonometry and area in diverse fields, from engineering and architecture to surveying and everyday life. Prepare to enhance your problem-solving skills, deepen your conceptual understanding, and discover the power of these mathematical tools.
Trigonometry Concepts
Trigonometry is a branch of mathematics that deals with the relationships between the sides and angles of triangles. It has a wide range of applications in various fields, including surveying, navigation, engineering, and astronomy.
The basic trigonometric functions are the sine, cosine, and tangent. These functions are defined as follows:
- The sine of an angle is the ratio of the length of the opposite side to the length of the hypotenuse.
- The cosine of an angle is the ratio of the length of the adjacent side to the length of the hypotenuse.
- The tangent of an angle is the ratio of the length of the opposite side to the length of the adjacent side.
These functions can be used to solve a variety of problems involving triangles. For example, they can be used to find the length of a side of a triangle, the measure of an angle, or the area of a triangle.
Relationship Between Angles and Trigonometric Ratios
The trigonometric ratios of an angle are determined by the angle itself. The following table shows the relationship between the angles and the trigonometric ratios:
| Angle | Sine | Cosine | Tangent |
|---|---|---|---|
| 0° | 0 | 1 | 0 |
| 30° | 1/2 | √3/2 | 1/√3 |
| 45° | √2/2 | √2/2 | 1 |
| 60° | √3/2 | 1/2 | √3 |
| 90° | 1 | 0 | ∞ |
As the angle increases, the sine and tangent functions increase, while the cosine function decreases.
Area Measurement: Practice 10-5 Trigonometry And Area
Area is a measure of the extent of a two-dimensional surface. It is defined as the number of square units that can fit inside a given shape. The SI unit of area is the square meter (m 2). Other commonly used units of area include the square centimeter (cm 2), the square kilometer (km 2), and the acre.
The area of a shape can be calculated using a variety of formulas. The most common formulas are:
- Triangle: A= (1/2) bh, where bis the base and his the height.
- Rectangle: A= lw, where lis the length and wis the width.
- Circle: A= π r2, where ris the radius.
Area is used in a variety of real-world applications, such as:
- Calculating the amount of paint needed to cover a wall.
- Determining the size of a building lot.
- Measuring the area of a forest.
Practice Problems
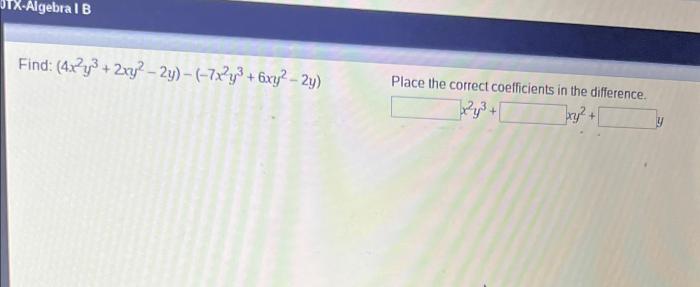
To enhance understanding and proficiency in trigonometry and area calculations, practice problems are essential. These problems provide an opportunity to apply theoretical concepts to practical scenarios, fostering a deeper comprehension of the subject matter.
The following section presents a set of practice problems that encompass both trigonometry and area calculations. Step-by-step solutions are provided, along with explanations that elucidate the reasoning behind each step. Additionally, an interactive table is included, allowing users to input their own values and receive immediate feedback on their calculations.
Practice Problems
- Problem 1:A right triangle has a base of 6 cm and a height of 8 cm. Calculate the area of the triangle and the length of the hypotenuse.
- Problem 2:A circle has a radius of 5 cm. Calculate the area and circumference of the circle.
- Problem 3:A rectangular garden has a length of 10 m and a width of 5 m. Calculate the area of the garden and the length of the diagonal.
Interactive Table
The following interactive table allows users to input their own values and receive immediate feedback on their calculations:
| Shape | Formula | Input Values | Result |
|---|---|---|---|
| Triangle | Area = (1/2)
|
||
| Circle | Area = π
|
||
| Rectangle | Area = length
|
Applications in Real-World Scenarios
Trigonometry and area calculations find widespread applications in various real-world scenarios. These concepts are instrumental in fields such as engineering, architecture, and surveying, enabling professionals to solve practical problems and design structures.
Engineering
In engineering, trigonometry is used to determine the forces acting on structures, calculate the strength of materials, and design efficient machines. Area calculations are crucial for determining the surface area of objects, which is essential for heat transfer, fluid flow, and other engineering applications.
Architecture
Architects rely on trigonometry to calculate angles, determine the slope of roofs, and design aesthetically pleasing structures. Area calculations are used to determine the floor space, wall area, and volume of buildings, which are critical for planning and cost estimation.
Surveying, Practice 10-5 trigonometry and area
Surveying involves measuring and mapping land areas. Trigonometry is used to calculate distances, angles, and elevations, while area calculations are used to determine the size and shape of land parcels. These concepts are essential for land use planning, boundary determination, and construction projects.
Additional Examples
- Navigation: Trigonometry is used in navigation systems to calculate the distance and direction to a destination.
- Astronomy: Area calculations are used to determine the surface area of planets and stars.
- Medicine: Trigonometry is used in medical imaging to determine the angles of bones and joints.
Tips for Effective Practice
Effective practice is essential for mastering trigonometry and area concepts. Regular review, repetition, and problem-solving are key strategies to enhance understanding and improve performance.
Independent Practice
- Review class notes and textbooks regularly to reinforce concepts.
- Solve practice problems from textbooks and online resources to apply your knowledge.
- Create flashcards or summary sheets to test your understanding of formulas and definitions.
Collaborative Learning
- Form study groups with classmates to discuss concepts, solve problems, and quiz each other.
- Participate in online forums or discussion boards to connect with other students and seek clarification.
- Attend review sessions led by instructors or tutors to ask questions and get additional support.
Staying Motivated
- Set realistic practice goals and track your progress to stay engaged.
- Reward yourself for completing practice sessions to maintain motivation.
- Find practice materials that are interesting and relevant to your interests.
FAQ Guide
What are the key concepts of trigonometry covered in practice 10-5?
Practice 10-5 focuses on the fundamental principles of trigonometry, including trigonometric functions (sine, cosine, tangent), the relationship between angles and trigonometric ratios, and the application of these concepts to problem-solving.
How does practice 10-5 help in understanding area measurement?
Practice 10-5 provides a comprehensive exploration of area measurement, encompassing the concept of area, its units of measurement, and the formulas for calculating the area of various shapes (e.g., triangles, rectangles, circles). Through practice problems and examples, students gain proficiency in applying these formulas to determine the area of different shapes.
What are the practical applications of trigonometry and area in real-world scenarios?
Trigonometry and area find widespread applications in diverse fields such as engineering, architecture, surveying, and everyday life. Engineers use trigonometry to calculate angles and distances in structural design, architects employ it in determining the dimensions and angles of buildings, and surveyors leverage it for land measurement and mapping.
In everyday life, trigonometry and area play a role in tasks like calculating the height of a tree or the volume of a container.